Extra Credit #2

Begins: Thursday, Feb 22, 2023

Due: Friday, Feb Mar 1, 2023, 3:15pm

The purpose of this assignment is to allow students to demonstrate their understanding of various abstract and applied physics topics. Because of the nature of this assignment, it will be graded fairly strictly—pay close attention to the Notes below. Assignments which are not completed as required will not be evaluated.

NOTES:

The Extra Credit Assignment consists of up to four problems, to be completed outside of class.
This assignment is Extra Credit and not required.

This assignment is intended to provide students with the opportunity to review some of the material we've covered, and not all students need the same amount of review. Students will be eligible to receive credit on problems completed according to the table below.

Your Current Test Average	Review Problems You Are Eligible to Complete
0 - 79%	Problems #1 - 4
80 - 89%	Problems #2 - 4
90 - 100%	Problems #3 - 4

The total points earned by a student on this assignment will be determined by the instructor based on a number of factors, including number of problems completed, difficulty of problem, and overall quality of assignments submitted by students.
You may discuss the problems with other people, but each person must complete his or her own work that will be turned in. Even when numeric answers to problems are the same, solutions from different students must be written independently, and differently, and developed and explained in each student's own words.
The assignment should have a separate cover sheet that includes your Name, Date, Course and Period, and Name of Assignment. Staple this cover sheet to the front of the problems that you've solved.
Each response must be hand-written on a separate piece of paper, which must include:
a) Your name and the problem number at the top of the page.
b) The original question written out in full.
c) Your solution, neatly hand-written, with all work shown in detail.
d) Drawings, diagrams, or graphs with labels that explain your solution more clearly.
e) Written explanations (blurbs, in English) explaining important steps in your solution.
f) The final answer, with a box around it.

See below for an example.

You're trying to impress the instructor with how well you can do. If in doubt, do a little more than you think you should, rather than trying to get by with less, and losing points on the problem.
The instructor will be available to answer questions about the assignment before school, after school, and occasionally in class. The instructor will not be able to help you if you leave all of your work until the night before the assignment is due.
Absolutely NO late work will be accepted for this assignment.

EXAMPLE PROBLEM AND SOLUTION:

SAMPLE PROBLEM:

In "The Matrix," Neo is given a test by Morpheus, who asks him to leap from the top of one building to another. Assume the buildings are each 100m tall, and 15m apart. Neo runs and leaps from the first building with a velocity of 5.00 m/s at an angle of 36.9 degrees (toward the second building).

a. Will he make it to the next building?
b. Where exactly will Neo land?

(Assume no air friction in this problem.)

Problems

1. Oh, crap

Examine the photo of a commercial toilet paper holder containing a large roll of toilet paper. The roll of paper has an inner radius a and an outer radius b.

Beginning from the standard equation for moment of inertia, derive the formula for moment of inertia for a cylinder of constant density, as a function of inner radius a, outer radius b and the density of the cylinder ρ.
Estimate the mass m of the entire roll based on your best reasonable guess of what such a roll of toilet paper would weigh.
Estimate the outer and inner radii b and a, based on your best reasonable guess.
Estimate the width of this roll of paper, based on your best reasonable guess or by measuring a real roll of toilet paper.
Calculate the actual moment of inertia I for this roll.
Assuming no friction, what amount of force would have to be applied at the end of the toilet paper in order to give the roll an angular acceleration of 5.0 rad/s²?
If the maximum force of tension that the toilet paper can withstand is 3.0 N before tearing, what is the maximum angular acceleration that the roll can have?
Estimate a reasonable size for a single piece of toilet paper for this roll.
Perform calculations to determine how many pieces of toilet paper are on this roll.

2. Beckman Institute Fountain

This problem asks you to take measurements to determine the velocity of the water in the "Gene Pool" fountain at the west end of the Beckman Auditorium on the Caltech campus. That fountain is currently not working, but perhaps you can solve the problem anyway.

a.) In the photo shown above, taken from near water level in order to minimize parallax, you can see a number of the fountain jets in operation, with varying angles of water streams. We'll be focusing on the jet in the foreground.

b.) In the photo shown below one can see the entire arc of the water's motion. The quality of the photo is such that you can (mostly?) see the two-inch tiles in the bottom of the pool, each of which are separated from its neighbor by ~2 millimeters of grout.

c.) Based on this information, perform calculations to identify

i. the horizontal distance of the water's trajectory

ii.) the maximum vertical height of the water

iii.) the water's velocity (magnitude and direction, or stated in unit vector notation) as it leaves the jet

3. The Meteoroid of 1992

Read this information about meteors, and examine this video of a fireball that was filmed by spectators at a New York football game on October 9, 1992. You can clearly see the fireball breaking up in the video. One of the pieces of the meteor survived the descent into the atmosphere and smashed into the trunk of a car.

Assume the meteoroid that made it to the ground had a mass equal to your mass in kg. Assume the meteroid encounters the Earth's atmosphere at a height of 250 km above the Earth's surface moving with a velocity of 12,000 m/s. To begin with, use the universal gravitation formula to determine the potential energy between the Earth and the meteoroid.
Calculate the total mechanical energy of the meteoroid at this height.
As the meteoroid falls toward the Earth's surface and through its atmosphere, explain what forces are acting on the meteoroid and whether those forces are increasing or decreasing, and why. (Assume that the satellite has not yet reached terminal velocity.)
Most of the meteoroid was burned up due to air friction R (this is a force) in the atmosphere, but a small chunk of it survived long enough to hit the car shown at the end of the video. Assume that the meteroid has reached an elevation where the force of earth's gravity is "mg," where g = 9.8 m/s/s. Use the Air Drag equation and reasonable values that you estimate for the mass m (make this YOUR mass) of the meteorite, the drag coefficient D (use D = 1), the cross-sectional area of the falling object (assume A = 0.040 m²), and air density ρ = 1.29 kg/m³ to:
1. determine a general equation for the Force of Air Resistance R as a function of velocity v
2. determine the terminal velocity of the meteorite as it falls though this air
3. derive an expression for the meteroid's velocity as a function of time assuming that at t = 0 it's velocity was 12,000 m/s.
Using the picture of the car as a guide, estimate the displacement, x, that the trunk of the car was dented in.
Calculate the average Force that the meteorite exerted on the car during the collision.
What happened to the meteoroid's total energy that it had before it entered the Earth's atmosphere? What happened to the part of the meteoroid that didn't make it to the earth's surface as a meteorite?

4. The Chain

A chain of length L is laid out stationary and in a straight line on the ground (you are looking down on it). The coefficient of kinetic friction between the chain and the surface is µ_k. Without lifting it up, a student grasps the chain at the left end and begins to drag it back along the chain's length. Note that in the beginning, there will only be a small part of the chain that has been doubled back and in motion along the ground, whereas toward the end most of the chain will be moving.

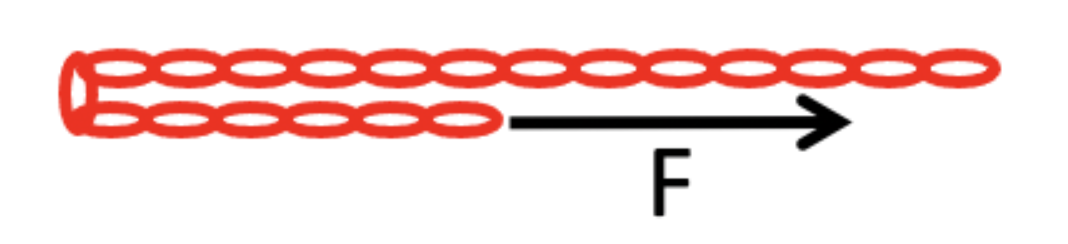
Draw an appropriate coordinate axis for this situation with the chain positioned as it will be after some arbitrary period of time. (Consider placing the origin at the left edge of the original chain.)
Assuming the mass M of the chain is uniformly distributed over the length of the chain, derive an expression for the work done by friction over the interval required to get the entire chain in motion.
If the force applied by the student is twice the frictional force applied, how fast will the chain be moving by the time the entire chain is in motion?
A second chain with a linear mass density of λ = kx replaces the original chain. Repeat Parts (a) and (b) for that situation, assuming the lighter end of the chain is on the left side.